


Next: About this document
Up: Status Update of PSI
Experiment R-89.01.1
Previous: Status Update of
PSI Experiment R-89.01.1
The experimental signature of a  event is determined by the prompt
decay
event is determined by the prompt
decay 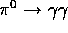 . The small branching ratio (
. The small branching ratio (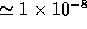 ) for the
) for the 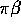 decay and the required high
measurement precision impose stringent requirements on the experimental
apparatus. The detector must be able to handle high event rates and cover
a large solid angle with high efficiency for
decay and the required high
measurement precision impose stringent requirements on the experimental
apparatus. The detector must be able to handle high event rates and cover
a large solid angle with high efficiency for 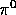 detection. Efficient
hardware suppression of background events calls for good energy and timing
resolution. At the same time the system must operate with low systematic
errors and be subject to accurate calibration.
detection. Efficient
hardware suppression of background events calls for good energy and timing
resolution. At the same time the system must operate with low systematic
errors and be subject to accurate calibration.
The Detector
We have chosen to detect decays at rest, and to use 

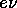 decays for
normalization. Consequently, our apparatus has the following main
components:
decays for
normalization. Consequently, our apparatus has the following main
components:
- (i)
- beam counters and a segmented active target to stop the pions,
- (ii)
- two concentric cylindrical multi-wire proportional chambers for
charged particle tracking surrounding the active target,
- (iii)
- a segmented fast veto counter surrounding the MWPCs,
- (iv)
- a high resolution segmented fast shower calorimeter
surrounding the active target and tracking detectors in a near-spherical
geometry, and
- (v)
- cosmic ray veto counters around the entire apparatus.
A schematic layout of the experimental apparatus is shown in Figs. 1
and 2.
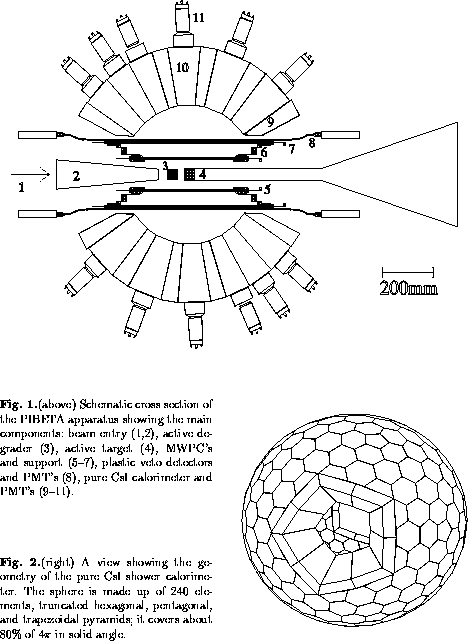
The spatial, energy and time resolution of the segmented shower calorimeter
are essential for efficient running at high rates. The PIBETA calorimeter
geometry calls for 240 truncated hexagonal and pentagonal pyramids; the
shapes were chosen for optimum light collection efficiency, and the
granularity to accommodate pion stop rates well in excess of 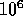 s
s . The calorimeter modules are made of pure CsI (for speed) and are
22 cm thick, which is equivalent to 12 radiation lengths. The outer radius
of the sphere is 48 cm. Efficient tracking of charged particles with good
double track resolution is necessary for a clean identification of the
. The calorimeter modules are made of pure CsI (for speed) and are
22 cm thick, which is equivalent to 12 radiation lengths. The outer radius
of the sphere is 48 cm. Efficient tracking of charged particles with good
double track resolution is necessary for a clean identification of the 

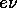 decay events -- hence the central tracking detectors.
decay events -- hence the central tracking detectors.
The Triggers
Selective bias-free triggers capable of handling high rates are an
essential requirement. We have designed fast analog hardware triggers
optimized to accept nearly all non-prompt 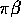 and
and 

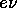 events
contained in the calorimeter with individual shower energy exceeding the
Michel endpoint (high threshold, HT
events
contained in the calorimeter with individual shower energy exceeding the
Michel endpoint (high threshold, HT 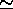 55 MeV), while
keeping the accidental rate to an acceptable level.
55 MeV), while
keeping the accidental rate to an acceptable level.
Each pion stopping in the target initiates a delayed pion gate (
DPG) not longer than 100 ns, delayed by about 10 ns in order to avoid
inclusion of prompt background events. Also generated are: (a) a short
gate ( PROMPT) coincident with the pion stop pulse, (b) a second
delayed pion gate ( DPG2) of the same duration as
DPG but started 100 ns later, (c) a short gate (COSM)
coincident with a cosmic muon passing through the veto housing, and a wide
unbiased gate (NOBG) overlapping the pion stop time. Event
triggers are generated on the basis of a coincidence of one of these gates
and shower signal(s) in the calorimeter. Gates other than the
DPG are necessary for a complete understanding of the background processes
and for the calorimeter gain monitoring and stabilization.
Pion beta decays are registered during the DPG through a
coincident detection of two 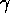 rays (from
rays (from 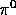

 )
in the shower calorimeter. The two photons are emitted nearly back-to-back
with about 67 MeV each. The positron is detected in the active target but
will not be included in the trigger, to avoid biasing it. The trigger
requires two localized showers occurring in opposing hemispheres of the
calorimeter, each exceeding the HT. In addition, there is a
low-threshold version ( LT
)
in the shower calorimeter. The two photons are emitted nearly back-to-back
with about 67 MeV each. The positron is detected in the active target but
will not be included in the trigger, to avoid biasing it. The trigger
requires two localized showers occurring in opposing hemispheres of the
calorimeter, each exceeding the HT. In addition, there is a
low-threshold version ( LT  2 MeV) of the same trigger
for background study purposes. Both the high- and low-threshold
2 MeV) of the same trigger
for background study purposes. Both the high- and low-threshold  triggers are replicated in the late DPG2 gate. The
low-threshold triggers are appropriately prescaled.
triggers are replicated in the late DPG2 gate. The
low-threshold triggers are appropriately prescaled.


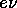 decays are identified by means of a dedicated trigger requiring a
localized single shower during the DPG with energy deposited in
the calorimeter exceeding HT. Due to the much higher branching
ratio, the
decays are identified by means of a dedicated trigger requiring a
localized single shower during the DPG with energy deposited in
the calorimeter exceeding HT. Due to the much higher branching
ratio, the 

 triggers are prescaled. As for the
triggers are prescaled. As for the  trigger, low- and high-threshold versions of the trigger are implemented
during both the DPG and the late DPG2 gates,
all suitably prescaled.
trigger, low- and high-threshold versions of the trigger are implemented
during both the DPG and the late DPG2 gates,
all suitably prescaled.
A good 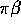 or
or 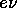 event is not allowed to be followed closely by
a beam particle in order to avoid pileup caused by a prompt event.
event is not allowed to be followed closely by
a beam particle in order to avoid pileup caused by a prompt event.
The proper working of the trigger relies on fast analog summing and
discrimination of the CsI module signals and an appropriate shower
clustering scheme. The size of the CsI crystal modules was chosen to match
approximately the characteristic lateral spreading of such showers, so that
a typical shower deposits most of its energy in three crystals sharing a
common vertex point. Hence, we sum over each of the vertices of the active
calorimeter, using overlapping summing regions for maximum trigger
efficiency. Analog summing and discrimination is performed in dedicated
linear summer/discriminator modules (UVA-125).
The basic energy summing regions, called clusters, sum over 9 detector
modules each, which is consistent with the planned pion stop rates below
10 s
s . Because clusters have to overlap, an individual crystal
contributes energy to up to three clusters using linear splitters
(UVA-126). There are 60 clusters in the entire calorimeter.
. Because clusters have to overlap, an individual crystal
contributes energy to up to three clusters using linear splitters
(UVA-126). There are 60 clusters in the entire calorimeter.
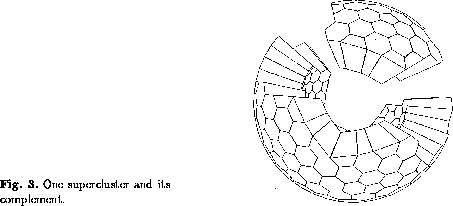
In order to simplify the trigger logic, the calorimeter is divided into 10
main regions called superclusters, each made up of a logical OR
of 6 overlapping clusters centered about a pentagonal shaped detector.
Each of the 10 superclusters has an associated opposing hemisphere
(complement) made up of the logical OR of the 5 superclusters
not neighboring it (cf. Fig. 3). Thus, for example, a 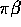 trigger
would require a coincidence of a given supercluster with its complement
inside the DPG gate.
trigger
would require a coincidence of a given supercluster with its complement
inside the DPG gate.
The analog energy summing method has been successfully tested in a 

 test measurement using a partial setup consisting of 26 CsI modules. Six
overlapping clusters were defined with up to 9 detectors each, and a
supercluster logical OR was formed. As described above,
test measurement using a partial setup consisting of 26 CsI modules. Six
overlapping clusters were defined with up to 9 detectors each, and a
supercluster logical OR was formed. As described above,
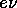 -LT and
-LT and 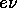 -HT triggers were formed.
A resulting energy spectrum is shown in Fig. 4.
-HT triggers were formed.
A resulting energy spectrum is shown in Fig. 4.
Instrumental Improvements
A significant new addition to the experiment is due to the recent
development by our PSI collaborators Ch. Brönnimann, R. Horisberger and
R. Schnyder of a fast (up to 800 MHz) inexpensive ``domino'' sampling chip
(DSC) to be used in conjunction with each calorimeter phototube. All
calorimeter and active target signals will be digitized. Using the
digitizer will help us achieve higher background pileup suppression and,
thus, improved calorimeter energy resolution at higher event rates. No
less important will be the improvement of the timing resolution due to the
sampling. Current estimates indicate that sub-100 ps rms accuracy in
determining the relative time of the leading edge of digitized CsI pulses
should be attainable. The final accuracy of the method will depend on the
noise level in the actual signal pulse shapes.
We had originally considered such a device for a more advanced stage of the
experiment. However, successful initial tests have made the chip available
sooner. Currently a dedicated PIBETA circuit board with zero suppression
and appropriate readout features is under development at PSI. The device
is about one to two years away from being fully integrated into the PIBETA
apparatus and routinely operational.
2. Backgrounds and Their Suppression
Background processes to be distinguished from  and
and  events fall into two groups: (a) prompt events due to the strong
interactions of the beam pions with any matter in their path, and (b)
delayed events due to other weak processes, i.e.,
events fall into two groups: (a) prompt events due to the strong
interactions of the beam pions with any matter in their path, and (b)
delayed events due to other weak processes, i.e.,  and
and 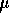 decays. Particularly important prompt background processes are pion
capture reactions followed by neutron and/or gamma emission. Among
the delayed background processes are the following decays (branching
ratios in parentheses):
decays. Particularly important prompt background processes are pion
capture reactions followed by neutron and/or gamma emission. Among
the delayed background processes are the following decays (branching
ratios in parentheses):
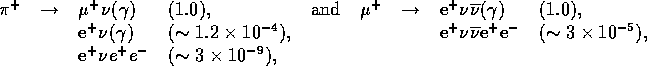
as well as their accidental coincidences.
Backgrounds from Beam Hadronic Interactions
Suppression of prompt backgrounds is necessary for obtaining clean spectra
of both 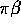 and
and 

 events. Hadronic interactions of the beam
pions in the active degrader (AD) and active target (AT) involve
scattering, charge exchange and nuclear reactions. All have been studied
in detail in our GEANT simulations. Main results of that analysis are
discussed in a separate ``PIBETA Note'' accessible at the PIBETA home
page.[1] We summarize here the essential points.
events. Hadronic interactions of the beam
pions in the active degrader (AD) and active target (AT) involve
scattering, charge exchange and nuclear reactions. All have been studied
in detail in our GEANT simulations. Main results of that analysis are
discussed in a separate ``PIBETA Note'' accessible at the PIBETA home
page.[1] We summarize here the essential points.
Hadronic scattering of pions in AT and AD leads to the same final outcome
as Coulomb scattering, namely, a delayed decay of the stopped pion.
Pion single charge exchange (SCX) in the AD and AT is the exclusive source
of hard prompt neutral showers with the branching ratio of about 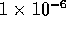 folded with our acceptance. Because they are prompt and are
vetoed efficiently by the delayed trigger gate, these events present a
problem only when they occur during an already open gate by virtue of an
undetected second beam pion charge exchanging in our beam detectors. A
suppression factor of 10
folded with our acceptance. Because they are prompt and are
vetoed efficiently by the delayed trigger gate, these events present a
problem only when they occur during an already open gate by virtue of an
undetected second beam pion charge exchanging in our beam detectors. A
suppression factor of 10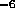 is required in order to bring this
background down to the level of 0.1% of the
is required in order to bring this
background down to the level of 0.1% of the 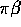 rate. This is
easily achieved by the beam counters B1 and AD, both of which are small
efficient plastic scintillators with optimized light readout. In addition,
the probability for a second beam pion is only 0.02 per beam bucket at the
beam rate of 10
rate. This is
easily achieved by the beam counters B1 and AD, both of which are small
efficient plastic scintillators with optimized light readout. In addition,
the probability for a second beam pion is only 0.02 per beam bucket at the
beam rate of 10 s
s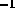 .
.
Charged ejectiles following pion hadronic interactions in the AD and AT
occur with the branching ratio of 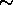 0.96% and deposit up to about 100
MeV in the calorimeter. Absence of a high-energy continuum background
characteristic for the prompt events in Fig. 4 shows that we have been able
to suppress it very well already in our test runs without having the
benefit of the full complement of charged particle tracking detectors.
0.96% and deposit up to about 100
MeV in the calorimeter. Absence of a high-energy continuum background
characteristic for the prompt events in Fig. 4 shows that we have been able
to suppress it very well already in our test runs without having the
benefit of the full complement of charged particle tracking detectors.
Backgrounds from Muon and Pion Decays
Since ours is a stopped experiment, the overwhelming source of background
are the muon decay events. The main triggers, 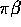 and
and


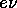 , both rely on discrimination (HT) to suppress
single Michel events. Thus, the main impact of muon decays on our
measurements is through accidental coincidences. The CsI spectra resulting
from the relevant processes are shown in Figs. 5--8 for the
, both rely on discrimination (HT) to suppress
single Michel events. Thus, the main impact of muon decays on our
measurements is through accidental coincidences. The CsI spectra resulting
from the relevant processes are shown in Figs. 5--8 for the 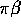 and
and


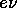 triggers, respectively. The rates and suppression factors
are listed below, in Tables 1 and 2 for the range of pion stop rates of
interest.
triggers, respectively. The rates and suppression factors
are listed below, in Tables 1 and 2 for the range of pion stop rates of
interest.
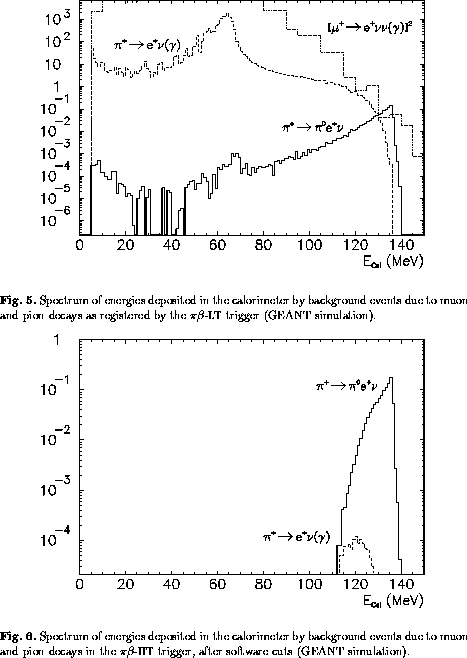
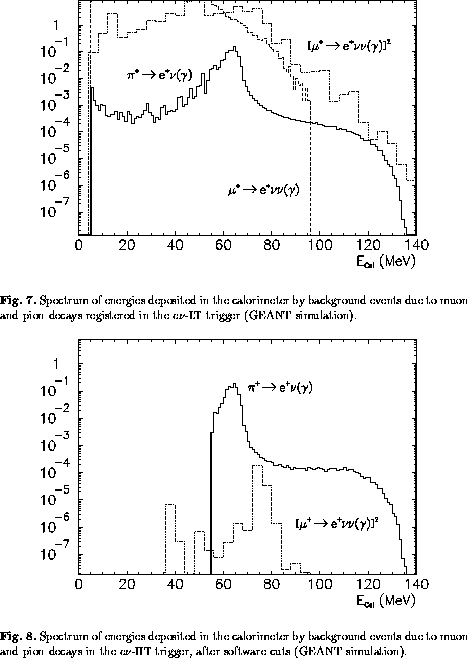
Of the pion decays, the radiative decay 

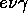 is the only
one with serious consequences for our data sample and systematics. On the
other hand, that problem is alleviated by the fact that the PIBETA detector
is highly suitable for the study of this decay. As shown in Figs. 5 and 6,
these events are well suppressed by off-line software cuts on colinearity
and charge particle counters.
is the only
one with serious consequences for our data sample and systematics. On the
other hand, that problem is alleviated by the fact that the PIBETA detector
is highly suitable for the study of this decay. As shown in Figs. 5 and 6,
these events are well suppressed by off-line software cuts on colinearity
and charge particle counters.
The more significant effect of the 

 events is on our
normalization. At our current level of Monte Carlo simulation we find that
our acceptance for the
events is on our
normalization. At our current level of Monte Carlo simulation we find that
our acceptance for the 

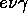 decay is approximately 98.9%
of a hypothetical pure
decay is approximately 98.9%
of a hypothetical pure 

 decay, making it a 1.1% correction. (The
measured branching ratio for the
decay, making it a 1.1% correction. (The
measured branching ratio for the 

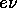 decay, to be used in our
normalization, includes the radiative decay.) We are further refining our
modeling of this decay, but are confident that we can produce a better than
10% uncertainty on this correction from both simulation and direct
measurement.
decay, to be used in our
normalization, includes the radiative decay.) We are further refining our
modeling of this decay, but are confident that we can produce a better than
10% uncertainty on this correction from both simulation and direct
measurement.
Summary of Rates and Suppression Factors
Since non-radiative decays are unphysical and do not occur in nature, we
have included the full treatment of the radiative decays of the pion and
muon in our Monte Carlo simulations. Neither in figures nor in the summary
tables below do we make the artificial separation of the nonradiative
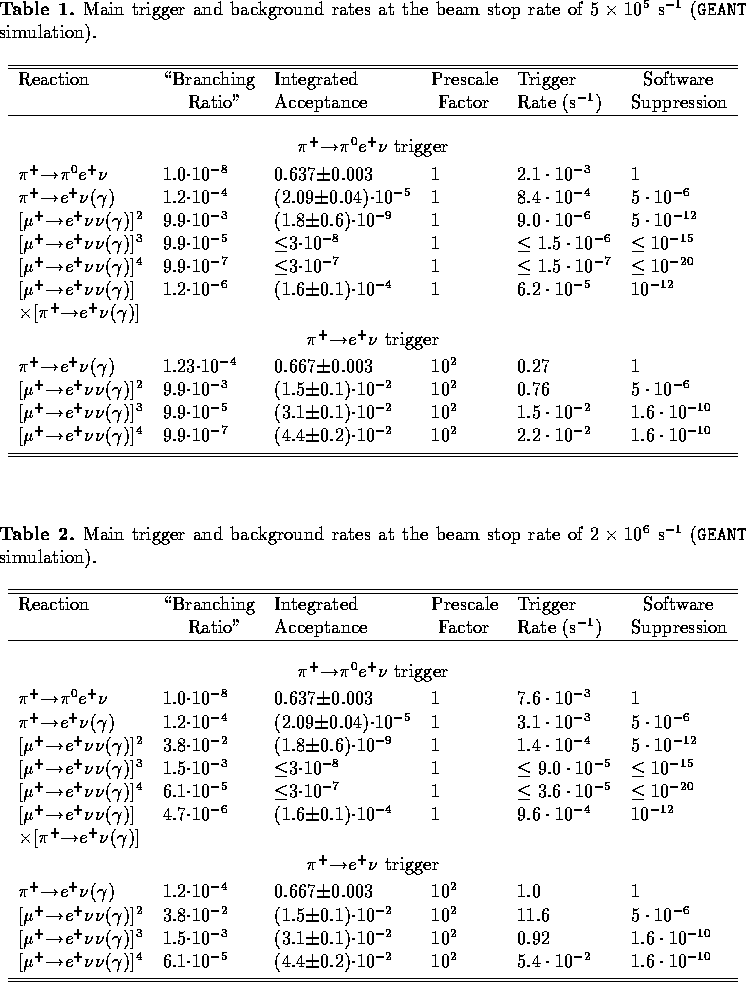
decays. The results presented in the Tables 1 and 2 below are to be taken
as work in progress, as we are continually improving our GEANT codes.
We present results of calculations at two beam stopping rates which bracket
our nominal running rate of  s
s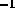 . The ``Branching Ratios''
column in the tables lists the physical branching ratios for the single
decay event types. In the case of accidental coincidences of several
decays, the ``branching ratios'' give the probability of that occurrence
given the stopping rate and the coincidence gate width.
. The ``Branching Ratios''
column in the tables lists the physical branching ratios for the single
decay event types. In the case of accidental coincidences of several
decays, the ``branching ratios'' give the probability of that occurrence
given the stopping rate and the coincidence gate width.
3. Measurement Uncertainties
The 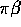 decay rate is evaluated from the ratio:
decay rate is evaluated from the ratio:
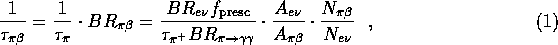
where 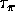 is the pion lifetime,
is the pion lifetime, 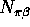 and
and 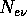 represent the numbers of good
represent the numbers of good 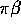 and
and 

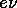 events,
respectively,
events,
respectively, 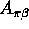 and
and 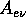 are the detector acceptances
for the
are the detector acceptances
for the 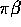 and
and 

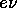 events, respectively, while
events, respectively, while 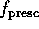 is
the the prescale factor for the
is
the the prescale factor for the 

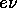 trigger.
trigger.
Due to the similarities between the two classes of events, the acceptances
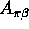 and
and 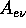 are nearly equal. At the same time both are
functions of many parameters:
are nearly equal. At the same time both are
functions of many parameters:
- (a) geometrical solid angle covered by the calorimeter including the
effects of:
- (i) lateral and axial extent of the pion stopping distribution,
- (ii) cracks between the CsI detector modules,
- (iii) alignment uncertainties;
- (b) lineshapes of the calorimeter detector modules' response to
photon- and positron-induced showers; details of the lineshapes are
affected by:
- (i) electromagnetic shower processes in CsI: leak-through, lateral
spreading, backsplash,
- (ii) electromagnetic interactions in the active target and
tracking detectors: bremsstrahlung, Bhabha scattering and in-flight
annihilation for the positrons, pair production, Compton scattering,
etc. for photons,
- (iii) volume uniformity of the CsI detector light response and
photoelectron statistics,
- (iv) gain stability of the calorimeter detector modules, and
- (v) photonuclear and electron knockout reactions in
CsI.
In addition, efficiencies of the charged particle detectors used in the
software cuts to remove the Michel and hadronic backgrounds from the final
data sample also affect the overall uncertainty.
We are continuing the study of these processes with increasing precision.
The important processes will be measured on-line and/or in dedicated
calibration runs, as well as simulated. A few less significant processes
can be reliably simulated with accuracy sufficient for our purposes. For
illustration, we show the GEANT simulated acceptances 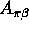 and
and
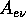 in Fig. 9 as functions of the cosine of the shower-reconstructed
polar angle
in Fig. 9 as functions of the cosine of the shower-reconstructed
polar angle 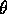 .
.
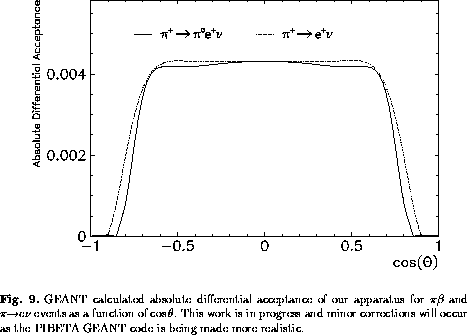
Integrated Absolute Acceptances
The shapes of the differential acceptance functions 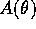 will, of
course, be precisely measured on-line in our experiment. However, absolute
normalization of these functions is necessary in order to obtain the
absolute integral acceptances appearing in Eq. (1). To this end we will
perform independent high-statistics measurements of the same quantities in
well controlled setups. Differential acceptance will be measured along
strips of constant azimuthal angle
will, of
course, be precisely measured on-line in our experiment. However, absolute
normalization of these functions is necessary in order to obtain the
absolute integral acceptances appearing in Eq. (1). To this end we will
perform independent high-statistics measurements of the same quantities in
well controlled setups. Differential acceptance will be measured along
strips of constant azimuthal angle  over the significant range of
over the significant range of
 in a section of the sphere using tagged positrons and photons.
Absolute measurements of
in a section of the sphere using tagged positrons and photons.
Absolute measurements of 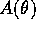 between
between 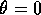 and
and 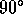 for single positrons and photons with about
for single positrons and photons with about 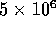 events each are
required to determine the ratio of the absolute integrated acceptances
events each are
required to determine the ratio of the absolute integrated acceptances
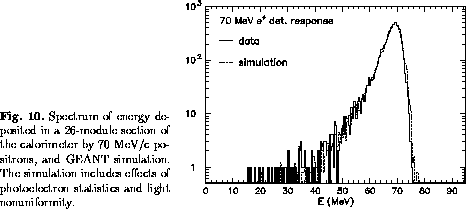
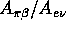 with an uncertainty of
with an uncertainty of 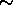 0.2--0.3% when
combined with other corrections. Here is how we plan to make the
measurements.
0.2--0.3% when
combined with other corrections. Here is how we plan to make the
measurements.
Positron Response We will tune the PSI  E1 beamline
for 70 MeV/c positrons, producing a low-intensity defocused beam on the
face of the CsI crystals. The positrons will be momentum-analyzed and
additionally identified by their time of flight with respect to the 50 MHz
accelerator RF pulse. Positron trajectories will be tracked by means of
thin wire chamber detectors. In this way we can illuminate evenly a large
section of the CsI calorimeter at once and obtain relatively quickly a
detailed position-dependent event-by-event calorimeter response. One
result of a test run without beam momentum analysis is shown in Fig. 10,
and compares favorably with GEANT simulation.
E1 beamline
for 70 MeV/c positrons, producing a low-intensity defocused beam on the
face of the CsI crystals. The positrons will be momentum-analyzed and
additionally identified by their time of flight with respect to the 50 MHz
accelerator RF pulse. Positron trajectories will be tracked by means of
thin wire chamber detectors. In this way we can illuminate evenly a large
section of the CsI calorimeter at once and obtain relatively quickly a
detailed position-dependent event-by-event calorimeter response. One
result of a test run without beam momentum analysis is shown in Fig. 10,
and compares favorably with GEANT simulation.
Our simulation of in-flight annihilations and scattering will be calibrated
by inserting plastic scintillator material of variable thickness in the
positron beam in front of the CsI. A thin plastic scintillation counter
will be used to determine the number of falsely neutral showers. These
measurements will be carried out with high statistics in order to enable
stringent off-line cuts and clean data samples.
The same set of measurements will be repeated for lower positron momenta in
order to calibrate the calorimeter response for the Michel positrons.
Gamma Response An analogous program of measurements
will be carried out with photon-induced showers. For this purpose, we
shall use a stopped 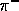 beam in a liquid hydrogen target, using the
reaction
beam in a liquid hydrogen target, using the
reaction 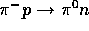 . The
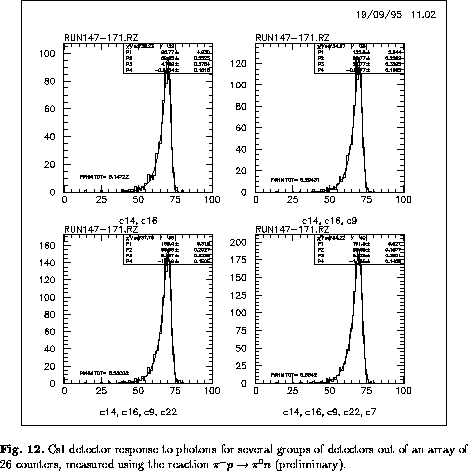
. The 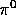 's in the above reaction carry
some 2.9 MeV of kinetic energy, which results in a total energy range for
the two decay photons of
's in the above reaction carry
some 2.9 MeV of kinetic energy, which results in a total energy range for
the two decay photons of  54 -- 83 MeV. This range includes all of
the photon energies of interest for the pion beta decay measurement (65.6
-- 69.4 MeV). A desired photon energy from the above range can be selected
by appropriately positioning the neutron detector with respect to the
shower counter and using suitably positioned second photon ``tag''
counters. This method allows for a simultaneous mapping of several
positions on the shower counter with desired photon energies. A simplified
schematic layout of the detector arrangement is shown in Fig. 11.
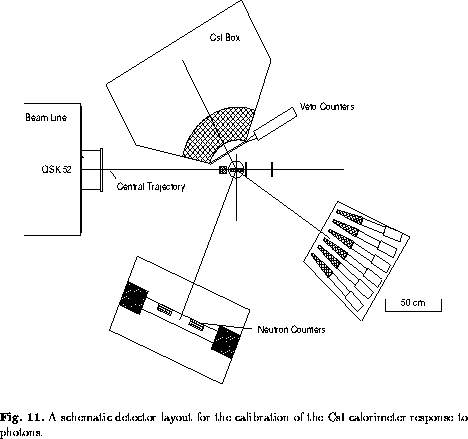
Preliminary results of a test run with a 26-counter CsI array using the
detector arrangement of Fig. 11 are shown in Fig. 12.
54 -- 83 MeV. This range includes all of
the photon energies of interest for the pion beta decay measurement (65.6
-- 69.4 MeV). A desired photon energy from the above range can be selected
by appropriately positioning the neutron detector with respect to the
shower counter and using suitably positioned second photon ``tag''
counters. This method allows for a simultaneous mapping of several
positions on the shower counter with desired photon energies. A simplified
schematic layout of the detector arrangement is shown in Fig. 11.
Preliminary results of a test run with a 26-counter CsI array using the
detector arrangement of Fig. 11 are shown in Fig. 12.
As in the case of the positron response, we need to measure the effects of
the active target, tracking detectors and air on the photon acceptance
(pair creation, Compton scattering, photonuclear reactions, etc.) Again,
we will insert plastic scintillator material of variable thickness in front
of the CsI. Throughout the measurements, a thin plastic veto counter
will register the charged component of the shower backsplash producing
false vetos. The effects of the active target and tracking counters are
of the order of 1 -- 2% for both 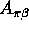 and
and  . The
planned counting statistics of over 10
. The
planned counting statistics of over 10 will ensure error bars in the
range of a few percent, i.e., well under 0.1% on the acceptances.
will ensure error bars in the
range of a few percent, i.e., well under 0.1% on the acceptances.
We note that both the photon and positron calibration data will reflect the
full extent of the photonuclear processes in CsI induced by electromagnetic
showers. Also folded in the same data will be the effects of photoelectron
statistics, nonuniformity of the detector light response, and cracks. The
CsI array to be calibrated will comprise about 1/4 of the sphere.
Deviations in the differential acceptance functions across the remaining
3/4 of the sphere will be determined from (a) the on-line 

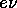 data for the
positrons where counting statistics is not a limiting factor, and (b) a
repeated calibration measurement of the
data for the
positrons where counting statistics is not a limiting factor, and (b) a
repeated calibration measurement of the  reaction with a
hydrogen target inside the sphere.
reaction with a
hydrogen target inside the sphere.
However, we wish to understand and simulate our acceptance functions in
detail, as a check that we have all above elements of the systematics under
control. To that end we have included in our GEANT code the effects of the
photonuclear reactions, photoelectron statistics, detector light
response nonuniformity and cracks, and are currently refining our treatment
of each of these effects.
Photonuclear reactions are added to GEANT on the basis of the published
compilations of cross sections[2]. The total probability for a 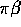 photon to undergo a photonuclear interaction outside the CsI calorimeter is
photon to undergo a photonuclear interaction outside the CsI calorimeter is
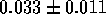 %, while the total probability of a photonuclear
interaction during a shower inside CsI is
%, while the total probability of a photonuclear
interaction during a shower inside CsI is 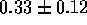 %. However, the
effect on the tail cutoff is further reduced, and there are additional
cancellations in the acceptance ratio
%. However, the
effect on the tail cutoff is further reduced, and there are additional
cancellations in the acceptance ratio 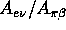 . Details of
the effect of photonuclear processes on the CsI lineshapes are presently
being refined.
. Details of
the effect of photonuclear processes on the CsI lineshapes are presently
being refined.
Our routine procedure for the acceptance or rejection of each CsI detector
module involves three concurrent measurements in a dedicated calibration
facility specifically set up for that purpose at PSI. The quantities
measured are: (i) the average number of photoelectrons/MeV, (ii) the optical
uniformity of the module's light response through a detailed cosmic muon
tomography of the detector volume, and (iii) the ratio of fast to the total
light output for minimum ionization particles. The complete detector
database will ensure an unambiguous folding of the detector response
parameters with the electromagnetic shower response.
Dynamic Gain Stabilization
Since our absolute acceptances involve an energy cutoff, properly
stabilized gains are crucial for keeping the uncertainties low. Besides
the usual sources of gain instability in the electronics and phototubes,
CsI itself has a pronounced temperature coefficient of light output of
about 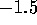 %/
%/ C. Given the drifts in ambient temperature in the
experimental hall, this would result in unacceptable shifts. Therefore the
entire detector system will be housed in a temperature stabilized enclosure
with temperature drifts regulated within 0.5
C. Given the drifts in ambient temperature in the
experimental hall, this would result in unacceptable shifts. Therefore the
entire detector system will be housed in a temperature stabilized enclosure
with temperature drifts regulated within 0.5 C.
C.
The overall gain of the system will be dynamically monitored and stabilized
by taking Michel events in the calorimeter with a rate of up to 200 Hz
during the entire experiment. The Michel spectrum of the muon decay falls
off rapidly around 50 MeV, producing a distinctive edge in each detector
very suitable for use in gain calibration. Using realistic spectra from
test runs we find for the uncertainty of the Michel edge position:
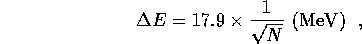
where N is the number of events in the Michel spectrum between 5 MeV and
50 MeV. To achieve, e.g., a gain calibration accuracy of 0.5 MeV at 50 MeV
(1%), a total of 1300 events are necessary. With the planned data
acquisition rate this can be done in about 10 seconds for the whole
detector. In other words, we can compensate a common gain drift of all
channels over very short time intervals. It takes about 50 minutes to
acquire the necessary statistics per channel in order to compensate the
gain drift of individual detector modules. Therefore, this method is well
suited for the individual PMT gain drifts which have time constants on the
order of hours.
Our calorimeter detectors run at a very low rate, under 10 pulses per
second. Nevertheless, after a careful study we have selected for use in
the calorimeter photomultiplier tubes with CsSb dynodes that display a much
higher degree of stability with respect to rate changes than the PMT's with
the ordinary BeCu dynodes. In addition, the PMT bases have been designed
for high linearity and gain stability vs. changes in load (see the PIBETA
experiment home page for details[1]).
pulses per
second. Nevertheless, after a careful study we have selected for use in
the calorimeter photomultiplier tubes with CsSb dynodes that display a much
higher degree of stability with respect to rate changes than the PMT's with
the ordinary BeCu dynodes. In addition, the PMT bases have been designed
for high linearity and gain stability vs. changes in load (see the PIBETA
experiment home page for details[1]).
In summary, taking  lineshapes and software energy cutoffs from our
test runs, we obtain an effect of 0.1% with a gain stabilization of 2.7%.
We expect to do better than that without major difficulties.
lineshapes and software energy cutoffs from our
test runs, we obtain an effect of 0.1% with a gain stabilization of 2.7%.
We expect to do better than that without major difficulties.
We finally note that the gain stabilization system is being designed to
operate fully automatically, without critical human intervention, in order
to ensure long-term stability.
Geometrical Uncertainties
The main source of uncertainty of geometrical nature in the absolute
acceptances 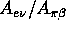 has to do with the position and spatial
spreading of the pion beam stopping distribution. Due to the cylindrical
symmetry of our detector system the uncertainties can be broken down into
the lateral and axial ones. Of the two, lateral uncertainties produce a
larger effect on the acceptances than the axial. Also, since the
has to do with the position and spatial
spreading of the pion beam stopping distribution. Due to the cylindrical
symmetry of our detector system the uncertainties can be broken down into
the lateral and axial ones. Of the two, lateral uncertainties produce a
larger effect on the acceptances than the axial. Also, since the
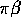 events require symmetrical coverage for the two photons emitted
in the
events require symmetrical coverage for the two photons emitted
in the 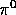 decay (nearly at rest),
decay (nearly at rest), 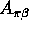 is affected far more
than
is affected far more
than 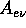 . We discuss here the dominant sources of uncertainty.
. We discuss here the dominant sources of uncertainty.
The ratio 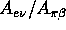 is reduced by approximately 0.34% per 1
mm of lateral displacement of the center of the beam stopping distribution
away from the central axis of the detector. This result was obtained using
the realistic pion stopping distributions measured in our test runs.
is reduced by approximately 0.34% per 1
mm of lateral displacement of the center of the beam stopping distribution
away from the central axis of the detector. This result was obtained using
the realistic pion stopping distributions measured in our test runs.
Analogously, increasing the width of the lateral size of the pion stopping
distribution by  mm, where
mm, where 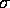 is the rms of the
distribution in the lateral direction, changes
is the rms of the
distribution in the lateral direction, changes 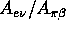 by
about 0.33%. For scale, we note that our measured pion stopping
distribution has a lateral rms of about 5 mm.
by
about 0.33%. For scale, we note that our measured pion stopping
distribution has a lateral rms of about 5 mm.
Thus both the centroid and the width of the pion stopping distribution have
to be monitored and controlled dynamically, as in the case of the gain of
the CsI detectors. The regular active target is segmented into 9 regions,
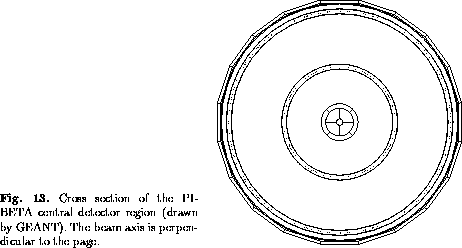
as shown in the GEANT drawing of the cross section of the central detector
region in Fig. 13. Prompt signals from each of the target segments will be
counted in a scaler, and the scalers read out every 10 s. The 5 central
detectors receive practically the entire stopped beam, each counting at
the same rate. The counting asymmetry (left--right or up--down) equals
2% for a shift of the centroid of 0.06 mm, corresponding to a 0.02% change
in the integrated acceptance ratio. This is an easily measurable asymmetry
given the counting statistics of 10 in 10 s, even after the Poisson
corrections for unresolved double hits and accidental coincidences with
Michel decays. Analysis of the measurement of the rms width of the
distribution shows that a 2% central--peripheral asymmetry corresponds to
a change in
in 10 s, even after the Poisson
corrections for unresolved double hits and accidental coincidences with
Michel decays. Analysis of the measurement of the rms width of the
distribution shows that a 2% central--peripheral asymmetry corresponds to
a change in  by 0.11 mm, or a 0.04% effect on the acceptance ratio
by 0.11 mm, or a 0.04% effect on the acceptance ratio
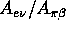 .
.
In addition, beam profiles will be monitored by active veto counters lining
the beam pipe just upstream of the degrader/target area. These counters
will not cut into the beam, but will detect the muon decay halo.
Techniques of stabilizing the beam geometry using differential counting
techniques have been implemented successfully by members of the PIBETA
collaboration in past experiments.
Periodically we will insert our 79-element fiber active target into the
apparatus in order to remeasure the shape of the stopping distribution.
In conclusion, we expect to control the acceptance systematics related to
the beam geometry at a level of better than 0.1%.
Timing Difference Between Showers Induced by Positrons and
Photons
A positron starts depositing energy in our calorimeter as soon as it reaches
the CsI detectors. Photons from pion beta decay, on the other hand,
interact initially with the detector once they are well inside it. Due to
this difference the DPG is effectively shifted between the two
processes. We have modeled the effect independently and in GEANT. After
discovering and correcting for a ``bug'' in the way GEANT processes the
shower particles on its stack, the results of the two methods have
converged at around 80 ps. We present here the GEANT result in Fig. 14.
Varying the discriminator threshold between 0.5 and 3 MeV produced a change
of less than 10 ps. We believe that we can pinpoint this correction to at
least 10% accuracy, or about 8 ps, which translates into a 0.03%
uncertainty on the 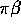 branching ratio.
branching ratio.

We also note that the timing difference will be measured on-line and can be
evaluated from our data to test our simulations. This is true because both
the 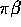 and
and 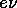 decays leave a (near) minimum ionizing positron
signal in the target that can be timed against the calorimeter. With the
help of the DSC digitizer and the correspondingly improved timing
resolution, we should be able to verify our Monte Carlo result.
decays leave a (near) minimum ionizing positron
signal in the target that can be timed against the calorimeter. With the
help of the DSC digitizer and the correspondingly improved timing
resolution, we should be able to verify our Monte Carlo result.
Detector Efficiences
The off-line analysis of our data will require software cuts for the
suppression of unwanted background events. Among others, this will involve
cuts on the charged particle counter signals. Thus the final yield will be
subject to the corrections for the inefficiencies of these detectors.
Inspection of Fig. 13 shows that both of the MWPC's and the plastic veto
counters are positioned in such a way that their efficiencies are measured
on-line throughout the experiment with very high statistics. Requiring in
the offline analysis that the target, all but the detector being
calibrated, and the CsI module(s) in the direct line of path of the
positrons produce signals will give a robust tag, allowing for
high-statistics continuous efficiency evaluation. Given prior experience
in such measurements we see no reason why we should not reach uncertainty
levels of the order of 10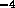 , rendering this source of error
unimportant.
, rendering this source of error
unimportant.
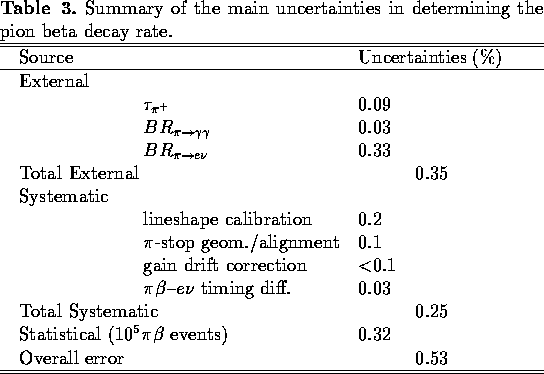
Summary of Uncertainties
The uncertainties of our planned experiment fall into three categories,
systematic, statistical, and errors on quantities not measured in our
experiment. They are all listed below in Table 3, with the respective error
limits indicated.
4. The Collaboration
The PIBETA experiment is an international collaboration of researchers from
7 groups (UVa, PSI/Univ. of Zürich, Arizona State Univ., Swierk, Dubna,
Tbilisi State Univ., and R. Boskovic Inst.) Each group has the
responsibility for one or more key parts of the apparatus. Equipment
funding has been secured in roughly equal parts from Swiss and
U.S. sources, with a considerable East European contribution in kind.
These funds are, for the most part, committed or already spent.
The question of manpower for this experiment has been a longstanding
concern. While all tasks are presently assigned, the experiment would
greatly benefit from a strengthening of the collaboration, given the large
volume of work ahead of us. The approximate current manpower commitments
from the various groups are (in real time, excluding teaching or
administrative duties):

5. The Timetable
Due to difficulties experienced by the suppliers of pure CsI modules, we
have been delayed with respect to our original plans. The extra time
was used for improvements in detectors, readout linearity, trigger
electronics, data acquisition, DSC digitizer development, etc.
Particularly beneficial was our development work on CsI detector surface
treatment coupled to the 3-dimensional cosmic muon tomography technique
developed specifically for this purpose, resulting in improved detector
energy resolution, consistently over a large sample of modules. All these
actions will eventually lead to shorter final commissioning time of the
apparatus and will serve to improve the quality of our data and expedite
``production'' measurements.
Current timetable for the experiment is as follows:
- 1996:
- all major detector components, all of the support structure
elements and control systems delivered and in place at PSI, all CsI
crystals delivered, tomographed and ready for installations by the
mid-year; assembly of apparatus in the fall;
- 1997:
- apparatus fully assembled at the beginning of the year;
proceed with detector shakedown and calibration; begin
 measurements at low pion stop rate;
measurements at low pion stop rate;
- 1998:
- ramp up to the nominal operating pion stop rate (
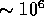 s
s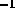 ); ``production'' runs;
); ``production'' runs;
- 1999:
- more ``production'' runs, likely into 2000
References
- The PIBETA home page is at URL: http://pibeta.psi.ch
(Europe), or http://helena.phys.virginia.edu/
 pibeta
(America).
pibeta
(America).
- J. Ahrens et al., Nucl. Phys. A251 (1975) 478;
A. Lepretre et al., Nucl. Phys. A367 (1981) 237;
H. Hebach, A. Wortberg, and M. Gari, Nucl. Phys. A267 (1076) 425;
B. L. Berman et al., Phys. Rev. 177 (1969) 1745;
R. L. Bramblett et al., Phys. Rev. 148 (1966) 1198;
G. G. Jonsson and B. Forkman, Nucl. Phys. A107 (1968) 52.



Next: About this document
Up: Status Update of PSI
Experiment R-89.01.1
Previous: Experimental Method
Bernward Krause
Mon Jan 29 08:39:51 MET 1996
 event is determined by the prompt
decay
event is determined by the prompt
decay 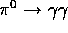 . The small branching ratio (
. The small branching ratio (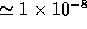 ) for the
) for the 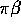 decay and the required high
measurement precision impose stringent requirements on the experimental
apparatus. The detector must be able to handle high event rates and cover
a large solid angle with high efficiency for
decay and the required high
measurement precision impose stringent requirements on the experimental
apparatus. The detector must be able to handle high event rates and cover
a large solid angle with high efficiency for 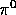 detection. Efficient
hardware suppression of background events calls for good energy and timing
resolution. At the same time the system must operate with low systematic
errors and be subject to accurate calibration.
detection. Efficient
hardware suppression of background events calls for good energy and timing
resolution. At the same time the system must operate with low systematic
errors and be subject to accurate calibration.





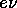 decays for
normalization. Consequently, our apparatus has the following main
components:
decays for
normalization. Consequently, our apparatus has the following main
components:

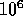 s
s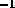 . The calorimeter modules are made of pure CsI (for speed) and are
22 cm thick, which is equivalent to 12 radiation lengths. The outer radius
of the sphere is 48 cm. Efficient tracking of charged particles with good
double track resolution is necessary for a clean identification of the
. The calorimeter modules are made of pure CsI (for speed) and are
22 cm thick, which is equivalent to 12 radiation lengths. The outer radius
of the sphere is 48 cm. Efficient tracking of charged particles with good
double track resolution is necessary for a clean identification of the 

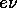 decay events -- hence the central tracking detectors.
decay events -- hence the central tracking detectors.
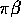 and
and 

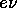 events
contained in the calorimeter with individual shower energy exceeding the
Michel endpoint (high threshold, HT
events
contained in the calorimeter with individual shower energy exceeding the
Michel endpoint (high threshold, HT 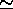 55 MeV), while
keeping the accidental rate to an acceptable level.
55 MeV), while
keeping the accidental rate to an acceptable level.
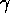 rays (from
rays (from 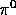

 )
in the shower calorimeter. The two photons are emitted nearly back-to-back
with about 67 MeV each. The positron is detected in the active target but
will not be included in the trigger, to avoid biasing it. The trigger
requires two localized showers occurring in opposing hemispheres of the
calorimeter, each exceeding the HT. In addition, there is a
low-threshold version ( LT
)
in the shower calorimeter. The two photons are emitted nearly back-to-back
with about 67 MeV each. The positron is detected in the active target but
will not be included in the trigger, to avoid biasing it. The trigger
requires two localized showers occurring in opposing hemispheres of the
calorimeter, each exceeding the HT. In addition, there is a
low-threshold version ( LT  2 MeV) of the same trigger
for background study purposes. Both the high- and low-threshold
2 MeV) of the same trigger
for background study purposes. Both the high- and low-threshold  triggers are replicated in the late DPG2 gate. The
low-threshold triggers are appropriately prescaled.
triggers are replicated in the late DPG2 gate. The
low-threshold triggers are appropriately prescaled.


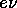 decays are identified by means of a dedicated trigger requiring a
localized single shower during the DPG with energy deposited in
the calorimeter exceeding HT. Due to the much higher branching
ratio, the
decays are identified by means of a dedicated trigger requiring a
localized single shower during the DPG with energy deposited in
the calorimeter exceeding HT. Due to the much higher branching
ratio, the 

 triggers are prescaled. As for the
triggers are prescaled. As for the  trigger, low- and high-threshold versions of the trigger are implemented
during both the DPG and the late DPG2 gates,
all suitably prescaled.
trigger, low- and high-threshold versions of the trigger are implemented
during both the DPG and the late DPG2 gates,
all suitably prescaled.
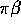 or
or 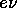 event is not allowed to be followed closely by
a beam particle in order to avoid pileup caused by a prompt event.
event is not allowed to be followed closely by
a beam particle in order to avoid pileup caused by a prompt event.
 s
s . Because clusters have to overlap, an individual crystal
contributes energy to up to three clusters using linear splitters
(UVA-126). There are 60 clusters in the entire calorimeter.
. Because clusters have to overlap, an individual crystal
contributes energy to up to three clusters using linear splitters
(UVA-126). There are 60 clusters in the entire calorimeter.
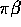 trigger
would require a coincidence of a given supercluster with its complement
inside the DPG gate.
trigger
would require a coincidence of a given supercluster with its complement
inside the DPG gate.



 test measurement using a partial setup consisting of 26 CsI modules. Six
overlapping clusters were defined with up to 9 detectors each, and a
supercluster logical OR was formed. As described above,
test measurement using a partial setup consisting of 26 CsI modules. Six
overlapping clusters were defined with up to 9 detectors each, and a
supercluster logical OR was formed. As described above,
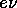 -LT and
-LT and 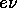 -HT triggers were formed.
A resulting energy spectrum is shown in Fig. 4.
-HT triggers were formed.
A resulting energy spectrum is shown in Fig. 4.

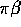 and
and 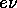 events fall into two groups: (a) prompt events due to the strong
interactions of the beam pions with any matter in their path, and (b)
delayed events due to other weak processes, i.e.,
events fall into two groups: (a) prompt events due to the strong
interactions of the beam pions with any matter in their path, and (b)
delayed events due to other weak processes, i.e.,  and
and 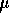 decays. Particularly important prompt background processes are pion
capture reactions followed by neutron and/or gamma emission. Among
the delayed background processes are the following decays (branching
ratios in parentheses):
decays. Particularly important prompt background processes are pion
capture reactions followed by neutron and/or gamma emission. Among
the delayed background processes are the following decays (branching
ratios in parentheses):
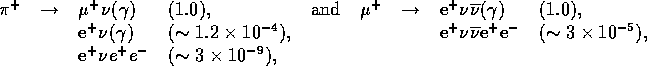
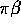 and
and 

 events. Hadronic interactions of the beam
pions in the active degrader (AD) and active target (AT) involve
scattering, charge exchange and nuclear reactions. All have been studied
in detail in our GEANT simulations. Main results of that analysis are
discussed in a separate ``PIBETA Note'' accessible at the PIBETA home
page.[1] We summarize here the essential points.
events. Hadronic interactions of the beam
pions in the active degrader (AD) and active target (AT) involve
scattering, charge exchange and nuclear reactions. All have been studied
in detail in our GEANT simulations. Main results of that analysis are
discussed in a separate ``PIBETA Note'' accessible at the PIBETA home
page.[1] We summarize here the essential points.
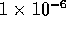 folded with our acceptance. Because they are prompt and are
vetoed efficiently by the delayed trigger gate, these events present a
problem only when they occur during an already open gate by virtue of an
undetected second beam pion charge exchanging in our beam detectors. A
suppression factor of 10
folded with our acceptance. Because they are prompt and are
vetoed efficiently by the delayed trigger gate, these events present a
problem only when they occur during an already open gate by virtue of an
undetected second beam pion charge exchanging in our beam detectors. A
suppression factor of 10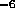 is required in order to bring this
background down to the level of 0.1% of the
is required in order to bring this
background down to the level of 0.1% of the 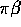 rate. This is
easily achieved by the beam counters B1 and AD, both of which are small
efficient plastic scintillators with optimized light readout. In addition,
the probability for a second beam pion is only 0.02 per beam bucket at the
beam rate of 10
rate. This is
easily achieved by the beam counters B1 and AD, both of which are small
efficient plastic scintillators with optimized light readout. In addition,
the probability for a second beam pion is only 0.02 per beam bucket at the
beam rate of 10 s
s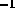 .
.
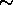 0.96% and deposit up to about 100
MeV in the calorimeter. Absence of a high-energy continuum background
characteristic for the prompt events in Fig. 4 shows that we have been able
to suppress it very well already in our test runs without having the
benefit of the full complement of charged particle tracking detectors.
0.96% and deposit up to about 100
MeV in the calorimeter. Absence of a high-energy continuum background
characteristic for the prompt events in Fig. 4 shows that we have been able
to suppress it very well already in our test runs without having the
benefit of the full complement of charged particle tracking detectors.
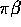 and
and


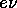 , both rely on discrimination (HT) to suppress
single Michel events. Thus, the main impact of muon decays on our
measurements is through accidental coincidences. The CsI spectra resulting
from the relevant processes are shown in Figs. 5--8 for the
, both rely on discrimination (HT) to suppress
single Michel events. Thus, the main impact of muon decays on our
measurements is through accidental coincidences. The CsI spectra resulting
from the relevant processes are shown in Figs. 5--8 for the 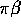 and
and


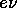 triggers, respectively. The rates and suppression factors
are listed below, in Tables 1 and 2 for the range of pion stop rates of
interest.
triggers, respectively. The rates and suppression factors
are listed below, in Tables 1 and 2 for the range of pion stop rates of
interest.




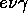 is the only
one with serious consequences for our data sample and systematics. On the
other hand, that problem is alleviated by the fact that the PIBETA detector
is highly suitable for the study of this decay. As shown in Figs. 5 and 6,
these events are well suppressed by off-line software cuts on colinearity
and charge particle counters.
is the only
one with serious consequences for our data sample and systematics. On the
other hand, that problem is alleviated by the fact that the PIBETA detector
is highly suitable for the study of this decay. As shown in Figs. 5 and 6,
these events are well suppressed by off-line software cuts on colinearity
and charge particle counters.


 events is on our
normalization. At our current level of Monte Carlo simulation we find that
our acceptance for the
events is on our
normalization. At our current level of Monte Carlo simulation we find that
our acceptance for the 

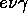 decay is approximately 98.9%
of a hypothetical pure
decay is approximately 98.9%
of a hypothetical pure 

 decay, making it a 1.1% correction. (The
measured branching ratio for the
decay, making it a 1.1% correction. (The
measured branching ratio for the 

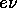 decay, to be used in our
normalization, includes the radiative decay.) We are further refining our
modeling of this decay, but are confident that we can produce a better than
10% uncertainty on this correction from both simulation and direct
measurement.
decay, to be used in our
normalization, includes the radiative decay.) We are further refining our
modeling of this decay, but are confident that we can produce a better than
10% uncertainty on this correction from both simulation and direct
measurement.

 s
s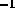 . The ``Branching Ratios''
column in the tables lists the physical branching ratios for the single
decay event types. In the case of accidental coincidences of several
decays, the ``branching ratios'' give the probability of that occurrence
given the stopping rate and the coincidence gate width.
. The ``Branching Ratios''
column in the tables lists the physical branching ratios for the single
decay event types. In the case of accidental coincidences of several
decays, the ``branching ratios'' give the probability of that occurrence
given the stopping rate and the coincidence gate width.
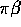 decay rate is evaluated from the ratio:
decay rate is evaluated from the ratio:
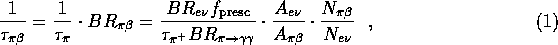
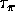 is the pion lifetime,
is the pion lifetime, 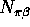 and
and 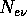 represent the numbers of good
represent the numbers of good 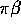 and
and 

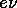 events,
respectively,
events,
respectively, 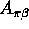 and
and 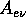 are the detector acceptances
for the
are the detector acceptances
for the 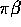 and
and 

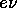 events, respectively, while
events, respectively, while 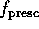 is
the the prescale factor for the
is
the the prescale factor for the 

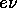 trigger.
trigger.
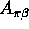 and
and 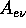 are nearly equal. At the same time both are
functions of many parameters:
are nearly equal. At the same time both are
functions of many parameters:
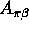 and
and
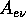 in Fig. 9 as functions of the cosine of the shower-reconstructed
polar angle
in Fig. 9 as functions of the cosine of the shower-reconstructed
polar angle 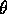 .
.

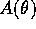 will, of
course, be precisely measured on-line in our experiment. However, absolute
normalization of these functions is necessary in order to obtain the
absolute integral acceptances appearing in Eq. (1). To this end we will
perform independent high-statistics measurements of the same quantities in
well controlled setups. Differential acceptance will be measured along
strips of constant azimuthal angle
will, of
course, be precisely measured on-line in our experiment. However, absolute
normalization of these functions is necessary in order to obtain the
absolute integral acceptances appearing in Eq. (1). To this end we will
perform independent high-statistics measurements of the same quantities in
well controlled setups. Differential acceptance will be measured along
strips of constant azimuthal angle  over the significant range of
over the significant range of
 in a section of the sphere using tagged positrons and photons.
Absolute measurements of
in a section of the sphere using tagged positrons and photons.
Absolute measurements of 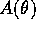 between
between 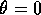 and
and 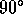 for single positrons and photons with about
for single positrons and photons with about 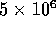 events each are
required to determine the ratio of the absolute integrated acceptances
events each are
required to determine the ratio of the absolute integrated acceptances
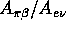 with an uncertainty of
with an uncertainty of 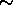 0.2--0.3% when
combined with other corrections. Here is how we plan to make the
measurements.
0.2--0.3% when
combined with other corrections. Here is how we plan to make the
measurements.
 E1 beamline
for 70 MeV/c positrons, producing a low-intensity defocused beam on the
face of the CsI crystals. The positrons will be momentum-analyzed and
additionally identified by their time of flight with respect to the 50 MHz
accelerator RF pulse. Positron trajectories will be tracked by means of
thin wire chamber detectors. In this way we can illuminate evenly a large
section of the CsI calorimeter at once and obtain relatively quickly a
detailed position-dependent event-by-event calorimeter response. One
result of a test run without beam momentum analysis is shown in Fig. 10,
and compares favorably with GEANT simulation.
E1 beamline
for 70 MeV/c positrons, producing a low-intensity defocused beam on the
face of the CsI crystals. The positrons will be momentum-analyzed and
additionally identified by their time of flight with respect to the 50 MHz
accelerator RF pulse. Positron trajectories will be tracked by means of
thin wire chamber detectors. In this way we can illuminate evenly a large
section of the CsI calorimeter at once and obtain relatively quickly a
detailed position-dependent event-by-event calorimeter response. One
result of a test run without beam momentum analysis is shown in Fig. 10,
and compares favorably with GEANT simulation.

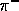 beam in a liquid hydrogen target, using the
reaction
beam in a liquid hydrogen target, using the
reaction 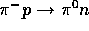 . The
. The 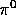 's in the above reaction carry
some 2.9 MeV of kinetic energy, which results in a total energy range for
the two decay photons of
's in the above reaction carry
some 2.9 MeV of kinetic energy, which results in a total energy range for
the two decay photons of  54 -- 83 MeV. This range includes all of
the photon energies of interest for the pion beta decay measurement (65.6
-- 69.4 MeV). A desired photon energy from the above range can be selected
by appropriately positioning the neutron detector with respect to the
shower counter and using suitably positioned second photon ``tag''
counters. This method allows for a simultaneous mapping of several
positions on the shower counter with desired photon energies. A simplified
schematic layout of the detector arrangement is shown in Fig. 11.
Preliminary results of a test run with a 26-counter CsI array using the
detector arrangement of Fig. 11 are shown in Fig. 12.
54 -- 83 MeV. This range includes all of
the photon energies of interest for the pion beta decay measurement (65.6
-- 69.4 MeV). A desired photon energy from the above range can be selected
by appropriately positioning the neutron detector with respect to the
shower counter and using suitably positioned second photon ``tag''
counters. This method allows for a simultaneous mapping of several
positions on the shower counter with desired photon energies. A simplified
schematic layout of the detector arrangement is shown in Fig. 11.
Preliminary results of a test run with a 26-counter CsI array using the
detector arrangement of Fig. 11 are shown in Fig. 12.


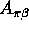 and
and  . The
planned counting statistics of over 10
. The
planned counting statistics of over 10 will ensure error bars in the
range of a few percent, i.e., well under 0.1% on the acceptances.
will ensure error bars in the
range of a few percent, i.e., well under 0.1% on the acceptances.


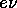 data for the
positrons where counting statistics is not a limiting factor, and (b) a
repeated calibration measurement of the
data for the
positrons where counting statistics is not a limiting factor, and (b) a
repeated calibration measurement of the  reaction with a
hydrogen target inside the sphere.
reaction with a
hydrogen target inside the sphere.
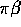 photon to undergo a photonuclear interaction outside the CsI calorimeter is
photon to undergo a photonuclear interaction outside the CsI calorimeter is
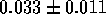 %, while the total probability of a photonuclear
interaction during a shower inside CsI is
%, while the total probability of a photonuclear
interaction during a shower inside CsI is 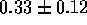 %. However, the
effect on the tail cutoff is further reduced, and there are additional
cancellations in the acceptance ratio
%. However, the
effect on the tail cutoff is further reduced, and there are additional
cancellations in the acceptance ratio 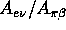 . Details of
the effect of photonuclear processes on the CsI lineshapes are presently
being refined.
. Details of
the effect of photonuclear processes on the CsI lineshapes are presently
being refined.
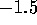 %/
%/ C. Given the drifts in ambient temperature in the
experimental hall, this would result in unacceptable shifts. Therefore the
entire detector system will be housed in a temperature stabilized enclosure
with temperature drifts regulated within 0.5
C. Given the drifts in ambient temperature in the
experimental hall, this would result in unacceptable shifts. Therefore the
entire detector system will be housed in a temperature stabilized enclosure
with temperature drifts regulated within 0.5 C.
C.
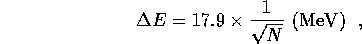
 pulses per
second. Nevertheless, after a careful study we have selected for use in
the calorimeter photomultiplier tubes with CsSb dynodes that display a much
higher degree of stability with respect to rate changes than the PMT's with
the ordinary BeCu dynodes. In addition, the PMT bases have been designed
for high linearity and gain stability vs. changes in load (see the PIBETA
experiment home page for details[1]).
pulses per
second. Nevertheless, after a careful study we have selected for use in
the calorimeter photomultiplier tubes with CsSb dynodes that display a much
higher degree of stability with respect to rate changes than the PMT's with
the ordinary BeCu dynodes. In addition, the PMT bases have been designed
for high linearity and gain stability vs. changes in load (see the PIBETA
experiment home page for details[1]).
 lineshapes and software energy cutoffs from our
test runs, we obtain an effect of 0.1% with a gain stabilization of 2.7%.
We expect to do better than that without major difficulties.
lineshapes and software energy cutoffs from our
test runs, we obtain an effect of 0.1% with a gain stabilization of 2.7%.
We expect to do better than that without major difficulties.
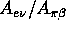 has to do with the position and spatial
spreading of the pion beam stopping distribution. Due to the cylindrical
symmetry of our detector system the uncertainties can be broken down into
the lateral and axial ones. Of the two, lateral uncertainties produce a
larger effect on the acceptances than the axial. Also, since the
has to do with the position and spatial
spreading of the pion beam stopping distribution. Due to the cylindrical
symmetry of our detector system the uncertainties can be broken down into
the lateral and axial ones. Of the two, lateral uncertainties produce a
larger effect on the acceptances than the axial. Also, since the
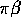 events require symmetrical coverage for the two photons emitted
in the
events require symmetrical coverage for the two photons emitted
in the 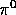 decay (nearly at rest),
decay (nearly at rest), 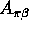 is affected far more
than
is affected far more
than 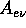 . We discuss here the dominant sources of uncertainty.
. We discuss here the dominant sources of uncertainty.
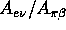 is reduced by approximately 0.34% per 1
mm of lateral displacement of the center of the beam stopping distribution
away from the central axis of the detector. This result was obtained using
the realistic pion stopping distributions measured in our test runs.
is reduced by approximately 0.34% per 1
mm of lateral displacement of the center of the beam stopping distribution
away from the central axis of the detector. This result was obtained using
the realistic pion stopping distributions measured in our test runs.
 mm, where
mm, where 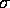 is the rms of the
distribution in the lateral direction, changes
is the rms of the
distribution in the lateral direction, changes 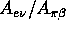 by
about 0.33%. For scale, we note that our measured pion stopping
distribution has a lateral rms of about 5 mm.
by
about 0.33%. For scale, we note that our measured pion stopping
distribution has a lateral rms of about 5 mm.
 in 10 s, even after the Poisson
corrections for unresolved double hits and accidental coincidences with
Michel decays. Analysis of the measurement of the rms width of the
distribution shows that a 2% central--peripheral asymmetry corresponds to
a change in
in 10 s, even after the Poisson
corrections for unresolved double hits and accidental coincidences with
Michel decays. Analysis of the measurement of the rms width of the
distribution shows that a 2% central--peripheral asymmetry corresponds to
a change in  by 0.11 mm, or a 0.04% effect on the acceptance ratio
by 0.11 mm, or a 0.04% effect on the acceptance ratio
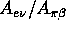 .
.

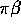 branching ratio.
branching ratio.

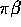 and
and 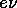 decays leave a (near) minimum ionizing positron
signal in the target that can be timed against the calorimeter. With the
help of the DSC digitizer and the correspondingly improved timing
resolution, we should be able to verify our Monte Carlo result.
decays leave a (near) minimum ionizing positron
signal in the target that can be timed against the calorimeter. With the
help of the DSC digitizer and the correspondingly improved timing
resolution, we should be able to verify our Monte Carlo result.
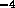 , rendering this source of error
unimportant.
, rendering this source of error
unimportant.


 measurements at low pion stop rate;
measurements at low pion stop rate;
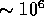 s
s ); ``production'' runs;
); ``production'' runs;
 pibeta
(America).
pibeta
(America).