One commences with an icosahedron, a polyhedron with twenty triangles and thirty-one great circles or geodesics. The great circles fall into three classes of six, fifteen and ten. The first class of six great circles can be seen by considering the twelve pairs of opposite vertices of the icosahedron. In addition, the icosahedron possesses fifteen pairs of opposite edges whose midpoints lead to the second class of fifteen great circles cutting perpendicularly through icosahedron edges. Finally, from the centers of the twenty triangles, ten pairs of opposite centers generate ten more great circles making the third class [Ken-76]. The great circles can be envisioned by assuming an axis going through a pair of opposite vertices, or mid points of opposite edges or centers of opposite faces, and imagining the icosahedron rotating around that axis.
An example of geodesic breakdown is as follows: starting with the family of six great circles, one outlines the faces of the icosahedron using only portions of the fifteen great circles.
Figure: One face (labeled 3) of the icosahedron
and its subdivisions are shown. The dashed lines (labeled 1) belong to the family of
the 6 great circles while the continuous lines (labeled 2) are portions of the 15 great
circles mapping out the faces of the icosahedron. Each edge is subdivided into
2 pieces according the two-frequency breakdown. In addition, the subdivisions
(line DE for instance) tend to run parallel to the icosahedron edges (line OA)
making this breakdown the class I type of subdivisions.
As a result, each icosahedron edge
is subdivided into two pieces and the process is called a two-frequency
breakdown. In addition, the subdivisions tend to run parallel to the
icosahedron edges and this is known as class I breakdown as shown in
figure 
.
As another example of geodesic breakdown, one begins with the fifteen great circles and simply removes the portions that outline the edges of the icosahedron. The result is also a two-frequency breakdown. However, contrary to the previous breakdown, this one has no complete great circles and the divisions run perpendicularly across icosahedron edges that have been removed: this is a class II breakdown.
The thirty-one great circles could be specified by a symmetry triangle.
Rotated and reflected a number of times, the symmetry triangle will reproduce
an icosahedron face as shown in figure where 4 rotations and
reflections of the symmetry triangle OCD will reproduce the icosahedron face
OAB. Therefore, 
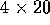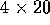such rotations and reflections will reproduces
the entire icosahedron with the family of six great circles and portions of
the fifteen. Any further subdivision made in the symmetry triangle will be
propagated throughout the whole icosahedron. Therefore, the breakdown process
starts with a triangular polyhedron face which is subdivided with a three-way
grid according to the breakdown frequency. The vertices of the grid are then
pushed outward until they are all at the same distance from the center of the
polyhedron (in the case of a spherical geometry). Then a set of rotations and
reflections will generate the whole sphere and some lines of the subdivisions
will map out the great circles of the icosahedron.